AbstractBackgroundThe lifetime risk of lung cancer incidence due to radiation for nonsmokers is overestimated because of the use of the average cancer baseline risk among a mixed population, including smokers. In recent years, the generalized multiplicative (GM)-excess relative risk (ERR) model has been developed in the life span study of atomic bomb survivors to consider the joint effect of radiation and smoking. Based on this background, this paper discusses the issues of radiation risk assessment considering smoking in two parts.
Materials and MethodsIn Part 1, we proposed a simple method of estimating the baseline risk for nonsmokers using current smoking data. We performed sensitivity analysis on baseline risk estimation to discuss the birth cohort effects. In Part 2, we applied the GM-ERR model for Japanese smokers to calculate lifetime attributable risk (LAR). We also performed a sensitivity analysis using other ERR models (e.g., simple additive (SA)-ERR model).
Results and DiscussionIn Part 1, the lifetime baseline risk from mixed population including smokers to nonsmokers decreased by 54% (44%–60%) for males and 24% (18%–29%) for females. In Part 2, comparison of LAR between SA- and GM-ERR models showed that if the radiation dose was ≤200 mGy or less, the difference between these ERR models was within the standard deviation of LAR due to the uncertainty of smoking information.
IntroductionThis study evaluates the lifetime risk of lung cancer incidence for nonsmokers after radiation exposure among a Japanese population. Currently, according to the International Commission on Radiological Protection (ICRP), the excess relative risk (ERR) model and the excess absolute risk model obtained by the Life Span Study (LSS) among atomic bomb survivors are used to evaluate cancer incidence or mortality risk in a target population [1]. Furthermore, the ICRP has used a hypothetical population to determine the average for the nominal risk factor. The nominal risk factor does not consider sex, age, or lifestyle (including smoking) [2].
In recent years, the National Aeronautics and Space Administration has conducted a cosmic radiation risk evaluation for astronauts using the baseline risk of nonsmokers because the use of the average United States population rate could result in overestimation of the radiation risk for nonsmokers [3]. In contrast, the impacts of the joint effect of smoking and radon have been discussed at work sites where radon exposure is expected [4]. Based on the background of the abovementioned research, it is necessary to consider not only the radiation exposure dose, age, and sex but also lifestyle factors such as smoking habits to evaluate the excess cancer risk after radiation exposure.
To evaluate the cancer risk due to radiation with smoking, this study investigated the effects of smoking on the evaluation of radiation risk for lung cancer in the Japanese population. Lung cancer is the most prevalent malignancy among the individual cancer risks in the Japanese population [5]. Other solid cancers have also been reported to be affected by smoking and radiation [6]. However, the risk evaluation of solid cancers other than lung cancer is excluded in this study because of the lack of the ERR model of smokers.
This study will be discussed in two parts. Part 1 estimates the cancer baseline rate of Japanese nonsmokers. Because the population-based cancer statistics in Japan [7] is intended for a mixed population without distinction of smokers and nonsmokers, conventional risk estimation methods for mixed populations may overestimate the radiation risk of nonsmokers. In this paper, the meaning of “baseline” was used as the cancer statistics of each non-radiation-exposed population for nonsmokers, smokers, and the mixed population of smokers and nonsmokers. This study estimates the baseline rate of nonsmokers from the baseline rate of mixed populations using smoking habit information and the ERR model in smokers. Moreover, a sensitivity analysis is conducted to examine the effects of changes in age on smoking rates and birth year effects.
Part 2 evaluates the impact of the joint effect of radiation and smoking on radiation risk assessment. A generalized multiplicative (GM)-ERR model of smoking and radiation was developed in the LSS [8–10]. This GM-ERR model was developed for use in atomic bomb survivors who were exposed to high doses in a short period of time. Although this model would be limited to apply to cases with low-dose and low-dose-rate exposure (e.g., nuclear workers), it may be useful to evaluate the risk due to radiation exposure and smoking. Furthermore, the attributable fraction (AF) and the population attributable fraction (PAF) of radiation and smoking risk are summarized. As a sensitivity analysis, the results of risk assessments between the GM-ERR model and other ERR models are compared, and the application of the GM-ERR model to low-dose radiation exposure situations is discussed. Finally, we comprehensively discuss about Part 1 and Part 2.
Materials and Methods1. Part 1 (Estimation of Nonsmokers Baseline Risk)This section explains our method of estimating the baseline rate for nonsmokers. Current common cancer baseline rate data in Japan do not distinguish between smokers and nonsmokers. Therefore, the radiation risk of nonsmokers may be overestimated when the averaged cancer baseline rate is used.
The evaluation models for nonsmokers’ baseline rate of lung cancer incidence are described by Equations (1)–(4). First, a simple estimation method of the baseline rate for nonsmokers was proposed using current smoking rate data without considering the birth cohort effect. The birth cohort effect is the variation in characteristics among individuals who are defined as a group of people born during a particular year and have differences in lifestyle, including smoking habits. The baseline rate of nonsmokers considering the birth cohort effect will be examined in a session of sensitivity analysis.
In Equation (1), the lung cancer incidence in all adults, defined as a mixed group of smokers and nonsmokers, can be calculated using the lung cancer incidence of nonsmokers, smokers, and smoking rates. Here adults aged >20 years were referred to as “all adults.” Equation (1) is a simple equation that considers only the current smoker information. The smoking effects that take into account past smokers (ex-smokers) in a population will be considered in the sensitivity analysis session.
where λm, is lung cancer incidence baseline rates in all adults; λs, lung cancer incidence baseline rates in smokers; λn, lung cancer incidence baseline rates in nonsmokers; SR, smoking rates in both smokers and nonsmokers; a, attained age; b, birth year (calendar year); c, cigarettes per day; and g, sex (male, female).
We next assumed that the lung cancer incidence rates in smokers can be calculated by multiplying the lung cancer incidence in nonsmokers and the ERR model of smokers, as in Equation (2). Equation (3) depicts the ERR model of smokers shown in the study of Cahoon et al. [10]. For the value of each parameter in Equation (3), the smoking parameters of the GM-ERR model [10] were used. In this simple estimation method, the parameter b representing birth effects among the parameters of the ERR model of smokers was fixed in 1915. This value of birth effects assumes that the age of the smokers was 30 years when the atomic bomb survivors were exposed. The impacts of the birth effects parameter on the baseline rate estimation in nonsmokers will be discussed in a sensitivity analysis session. In this paper, we assumed that smokers started smoking at the age of 20 years, as smoking is legalized in Japan at the age of 20 years.
where ERRs, is ERR model for smokers; φg, ERR per 50 pack-years (20 cigarettes per pack), 5.71 for male and 5.83 for female [10]; φb, birth cohort (change per decade decrease in birth year), 0.09 [10]; μ1, coefficient of log (duration/50), 1.09 [10]; μ2, coefficient of log (duration /50)2, −0.33 [10].
The ERR model of smokers represents smokers with different smoking habits, as represented by the number of cigarettes per day. The lung cancer incidence in nonsmokers can be calculated using Equation (4), substituting Equation (2) into Equation (1). Here, for cigarette per day “c” of the ERRs in Equation (4), the average value of each of the Japanese male and female smokers in Table 1 was used.
According to Equation (4), the estimated lung cancer incidence rates of nonsmokers depended not only on age and sex, but also on the smoking rate of smokers. In this study, the latest data regarding smoking habits, namely smoking rates, and number of cigarettes per day, for the Japanese population in 2017 were used [11, 12] (Table 1). In this paper, we define the Japanese population aged >20 years in 2017 as the target population for lung cancer risk assessment. The lung cancer incidence rates of the mixed population were based on data from the National Cancer Center in Japan. The cancer incidence rates in Japan in 2017 [5] were smoothed using spline interpolation based on an interval of 5 years to an interval of 1 year. The overall Japanese lung cancer incidence was 124,510 in 2017 [5]. Number of total samples of cigarettes per day were 912 males and 251 females [11].
The lifetime attributable risk (LAR) was used as a risk measure to compare the following radiation and smoking exposure scenarios [13]. The LAR approximates the risk of exposure-induced cases/deaths (REIC/REID) at low doses (few hundreds mSv). The LAR differs from the REIC/REID in that the survival function does not consider the number of persons dying from radiation-induced disease, thus the calculation of LAR is simpler than that of REIC/REID [14].
The dose and dose rate effective factor was not considered in this study due to the conservative estimation of radiation risk.
Equation (5) shows the LAR for population that started smoking and radiation exposure at the age of 20 years.
where ERR, is ERR model for population that started smoking and radiation exposure; S, probability of surviving at age a; λ, cancer incidence baseline rate for Japanese people per 10,000 persons; and D, radiation dose (mGy per year).
In this study, the estimation duration of LAR was set between 25 and 85 years considering the minimum latency period (5 years). The reason for the lower age limit (age=25 years) set for the integration of LAR was the start of smoking and radiation working and considering the minimum latency period of cancer. In contrast, the reason for the upper age limit (age=85 years) set for the integration of LAR was to remain in accordance with the baseline data of cancer incidence in Japan. Survival probabilities (S) were calculated using the latest Japanese life tables for the year 2017. Here, life tables indicate the probability that a person of that particular age will die before his or her next birthday for each age [15].
To compare the baseline cancer risk with LAR, the lifetime baseline risk (LBR) was defined according to the following Equation (6):
where LBR is the cancer incidence LBR for nonsmokers, all adults, and smokers.
2. Part 2 (Risk Assessment Considering the Joint Effect of Radiation and Smoking)1) Radiation risk modelPart 2 discusses the risk assessment of lung cancer incidence caused by radiation and smoking. Equation (7) shows the ERR model for single radiation exposure (ERRRs) as a function of attained age, sex, and annual radiation dose [10]. In this study, the ERR model of single radiation exposure was extended to chronic radiation exposure to evaluate annual radiation exposure (ERRRc). In Equation (8), the duration of the radiation exposure was fixed between the ages of 20 and 60 as a hypothetical working duration. Equation (8) represents the case where attained age exceeds the age at exposure, a>e. If age at exposure exceeds attained age, there is no cancer risk due to radiation exposure. Therefore, the value of ERRRc is zero in Equation (9). For the value of each parameter in Equations (7) and (8), the radiation parameters of the GM-ERR model [10] were used.
In case of ages, a>e,
In case of ages, e=>a,
where ERRRs, radiation ERR model for single exposure; ERRRc, radiation ERR model for chronic exposure; D, radiation dose (mGy per year); e, age at exposure; βg, ERR per Gy at the age of 30 years and attained age of 70 years, 0.34 for male and 1.32 for female [10]; θ, coefficient for effect modification by age at exposure, 16% [10]; and γ, coefficient for the modification due to attained age, −2.11 [10].
In the case of single exposure ERR, the LAR equation depends on the age at exposure e, as in Equation (10). In the case of ERR of chronic exposure, it becomes the same LAR equation as Equation (5).
2) GM-ERR modelThe GM-ERR model was used in this study to evaluate the joint effects of radiation and smoking for lung cancer incidence risk [10]. The smoking ERR model of Equation (3) and the radiation ERR model of Equation (7) or Equation (8) were used. In Part 2, we targeted the populations that do not consider the birth cohort φb and age at which smoking was quit (s).
This GM-ERR model has demonstrated better reproducibility of the risk of lung cancer incidence for smokers in the LSS cohort than the other ERR models [8–10]. Equation (11) depicts the GM-ERR model using radiation and smoking ERR models.
where ERRGM, GM-ERR interaction model; ERRs, smoking ERR model; ERRR, radiation ERR model; c, cigarettes per day; a, attained age; g, sex (male, female); and f, function of smoking variables.
In Equation (12), the radiation-smoking interaction of the GM-ERR model was expressed as a linear-quadratic function of cigarettes per day (c).
Results and Discussion1. Part 1 (Estimation of Nonsmokers’ Baseline Risk)1) Estimation of nonsmokers’ baseline risk of Japanese population
Fig. 1 shows the age-specific incidence rates of lung cancer among Japanese males and females in 2017 and those among nonsmoking Japanese males and females, respectively. The estimated cancer incidences of nonsmokers shown in Fig. 1 are the calculated results using Equation (4). Comparison of cancer incidence between all adults and nonsmokers at the age of 85 years indicated that the risk of lung cancer incidence decreased by 65% for males and 33% for females. The difference in the decreasing rates between all adults and nonsmokers was caused by the smoking rates.
There are few reports of lung cancer incidence among Japanese nonsmokers. In the present study, the observations of lung cancer incidence rates of nonsmoking Japanese females are shown in Fig. 1 for each attained age (nonsmoker females [observed]). A previous survey reported the lung cancer incidence rates of nonsmoking Japanese females in three prefectures [16]. In this study, to compare with the risk of lung cancer incidence in nonsmoking females, the results of the survey in the three prefectures were averaged at each attained age. As shown in Fig. 1, the estimated risk of lung cancer incidence in nonsmoking females is close to the average values of the observed values in the three prefectures. Unfortunately, the age trend of lung cancer incidence rates for nonsmoking Japanese males was not found. Therefore, rather than comparing the observed data, the validity of the baseline risk estimation for nonsmoker males was confirmed using the ratio of LAR between smokers and nonsmokers.
To validate the method of baseline risk estimation for nonsmokers, the results of the LBR of the estimated baseline risk of nonsmokers, all adults, and smokers are summarized in Fig. 2. The raw data for lifetime lung cancer incidence rates in the Japanese population were 10% for males and 5% for females in 2017 [7]. Here, the LBRs of lung cancer incidence of all adults were 9.5% for males and 4.3% for females. From these results, the LBR approximates the cancer incidence rates.
Next, the LBR of nonsmokers was estimated from the average and standard deviation of cigarettes per day of Japanese smokers in Table 1. The uncertainty of the LBR of the estimated nonsmokers and smokers was derived from the standard deviation of the number of cigarettes per day in the Japanese population.
The ratio of LBR between all adults and nonsmokers was 2.2 (range, 1.8–2.5) for males and 1.3 (range, 1.2–1.4) for females. In other words, the LBRs from all adults to nonsmokers decreased by 54% (range, 44%–60%) for males and 24% (range, 18%–29%) for females.
The risk ratios (RRs) of lifetime risk between smokers (LBR+LAR of smoking) and nonsmokers were 6.4 (range, 4.3–8.2) for males and 5.7 (range, 4.1–7.2) for females. From the Japanese cohort study [17], the RRs of lifetime lung cancer incidence among smokers and nonsmokers were 4.5 (range, 3.0–6.8) for males and 4.2 (range, 2.4–7.2) for females. The RRs of lifetime risk between smokers and nonsmokers estimated in this study and the observations in previous studies were consistent within the uncertainty of smoking information. These results suggest that the baseline risk estimation of nonsmokers using this simple estimation method was generally reasonable.
2) Sensitivity analysis: birth cohort effectsIn the previous session of Part 1, only current smoker information was considered to estimate the baseline risk of nonsmokers where information of past smokers (ex-smokers) was neglected.
In this session, a sensitivity analysis was performed on the baseline risk estimates for nonsmokers to evaluate the effects of birth cohort and ex-smokers. First, the birth year effect (parameter b in the smoking ERR model, Equation [3]) was considered. This implies that the ERR model was set in consideration of the age distribution of the population as of 2017. Next, the information of ex-smokers was used to define populations who smoked in the past but were not current smokers. This was considered to estimate the baseline risk for nonsmokers. For the ERR model of ex-smokers, the formula used in the study of Cahoon et al. [10] was used. The parameters of the ERR model for ex-smokers were set to be the same as the GM-ERR model (Table 3 of Cahoon et al. [10]).
Equation (13) shows the ERR model of ex-smokers for lung cancer incidence as functions of cigarettes per day (c), age at which smoking was quit (s), attained age (a), birth year (b), and sex (g) [10].
(13)where φg, is ERR per 50 packs per year (20 cigarettes per pack), 5.71 for male and 5.83 for female [10]; φb, birth cohort (change per decade decrease in birth year), 0.09 [10]; μ1, coefficient of log (duration/50), 1.09 [10]; μ2, coefficient of log (duration/50)2, −0.33 [10]; υ, power of years post-quitting + 1, −0.18 [10]; b, birth year (calendar year); and s, age at which smoking was quit.
Next, to estimate the baseline risk of nonsmokers considering the information of ex-smokers, the mixed population was divided into three groups, namely smokers, ex-smokers, and nonsmokers. Equation (1) was extended.
where λsq, is lung cancer incidence baseline rates in ex-smokers; QR, rates of quitting smoking for each age; and k, birth cohort.
The baseline risk of ex-smokers can be written as Equation (15) from Equation (2) of baseline risk of smokers. Then, by inserting Equation (14) into Equation (15), the baseline risk estimation equation for nonsmokers is extended to Equation (16). Here, for cigarettes per day “c” of the ERRs in Equation (13), the average value of each of the male and female smokers in Table 1 was used.
According to Equation (16), it was necessary to calculate the variation in the rates of quitting smoking for each age (QR) to estimate the baseline risk of nonsmokers. In Japan, smoking rates, particularly in males, have been decreasing since 1965 [12]. This has resulted in an increase of QR at the same age per calendar year. The calculation of QR is shown below. To obtain information of when Japanese ex-smokers quit smoking, the trend data on smoking rates by age of Japanese males and females were obtained every 10 years (Table 2). Second, the QR and smoking rates in each cohort of 2017 were examined over time, and the decreases in smoking rates were calculated. Based on these results, the QRs were calculated (Table 3). The data on age-specific smoking rates in the past were obtained from the Japan Health Promotion & Fitness Foundation’s website [12].
3) Results of sensitivity analysis considering the birth cohort effects and ex-smokers
Fig. 3 presents the LBR estimates for nonsmokers when the birth year effect and the ex-smokers were considered. Considering the birth year effect, the LBR of nonsmokers was slightly higher than that of this simple estimation method. However, the average value of LBR was within the error bar due to the standard deviation of the number of cigarettes per day. Next, considering ex-smokers, the LBR of nonsmokers was slightly lower than that of this simple method. However, the average value of LBR was within the error bar. Finally, when the birth year effect and ex-smokers were considered at the same time, the baseline risk for nonsmokers was almost the same as for this simple estimation method. From the abovementioned sensitivity analysis, it was found that the cohort effect (birth effect and ex-smokers) was less sensitive to the baseline risk estimation of nonsmokers than the information of cigarettes per day. No significant difference was detected in the baseline risk estimates of nonsmokers, even when the cohort effect was taken into consideration. Consequently, the radiation risk of smokers was evaluated using the estimated baseline risk of nonsmokers based on the current smoking information that was the simple estimation method from Part 2 of this paper.
In Part 1, the LBR estimates for nonsmokers was modeled and its validity was investigated considering the birth cohort effects and ex-smokers. In developing our model, we used the ERR model for the unexposed smokers in the LSS. This smoking ERR model is statistically described to project the smoking effects on the baseline rate of lung cancer incidence, and there is no other model that can provide the relationship of birth years with cigarettes per day, etc. The results of the sensitivity analysis indicated the practical validity of our model that can estimate the LBR estimates for nonsmokers.
2. Part 2 (Risk Assessment Considering the Joint Effect of Radiation and Smoking)1) Results of radiation riskIn this section, the LAR of radiation exposure without smoking was calculated to evaluate how the radiation risk of nonsmokers changed compared with the radiation risk for all adults. The radiation risks of lung cancer incidence for all adults and nonsmokers are shown in Fig. 4 and Table 4. To examine the extent to which the radiation risk for nonsmokers differed from the radiation risk for all adults, the radiation risks for all adults and nonsmokers were calculated for each age at exposure in males and females, respectively. Table 4 presents the results of the LAR of chronic exposure (2.5 mGy per year from the age of 20 to 60 years, a total lifetime dose of 100 mGy) that were calculated using Equation (8). The LAR of radiation exposure was higher in females than in males. This difference was due to the difference in the radiation ERR model parameters [10]. The difference in the ratio of radiation LAR between nonsmokers and smokers was greater in males than in females. This difference in the ratio of LAR was affected by the magnitude of the smoking rate of the group in the estimated baseline risk of nonsmokers (Table 1).
As shown in Fig. 4, the LAR value peaked around the age of 60 years. This is because the parameter θ coefficient for effect modification by age at exposure in the radiation ERR of lung cancer in Equation (7) is 16%, and therefore the value of ERR increases as the age at exposure increases. Moreover, according to Fig. 1, the lung cancer baseline risk increases with increasing age. In contrast, to calculate LAR, the survival curve S(e), which decreases as the attained age increases, is taken into consideration; therefore, the value of radiation lung cancer LAR decreased around the age of 60 years.
The LAR of chronic exposure totaling 100 mGy and the LAR of single exposure to 100 mGy at the age of 40 years were similar values because the LAR of the radiation exposure period from the age of 20 to 60 years increases monotonously. Therefore, this study used chronic exposure conditions to eliminate age-related risk fluctuations at the time of exposure.
2) Results of radiation and smoking riskIn this section, the radiation risk of lung cancer incidence in smokers was evaluated. The LARs of smokers exposed to differing radiation doses and number of cigarettes per day were evaluated. In a previous study [10], the highest value appeared at c=10 lifetime lung cancer risk per 1,000 mGy in atomic bomb survivors in Hiroshima and Nagasaki. This is the first study to apply the GM model to a population different from the LSS cohort.
Fig. 5 shows the LARs of Japanese male and female smokers according to Equations (3), (4), (5), (8), and (11) with different numbers of cigarettes per day and total radiation exposure dose (mGy). In the case of unexposed male (0 mGy) and exposed male (100–200 mGy) smokers, the LARs of smokers monotonically increased according to the number of cigarettes per day. These LARs have no peak value. In contrast, the LAR of exposed male smokers (300 mGy) increased until c<10. The LARs (300 mGy) were almost constant up to c=20 and increased when c>20. Moreover, the highest value was obtained at c=10 due to the joint effect of exposed male smokers (500 mGy).
As shown in Fig. 5, females who are smokers were at a higher risk of developing lung cancer due to radiation than males who are smokers. This is because females have a higher ERR per Gy than males (Table 3 of Cahoon et al. [10]). In addition, as shown in Fig. 5B, in the case of females exposed to 300 mGy, the highest LAR values are apparently close to c=10.
Although these observations that LARs (>300 mGy) decrease as the number of cigarettes per day increases from 10 to 25 appear to be counterintuitive, a similar pattern was found in the LSS among the atomic bomb survivors [8–10]. The LSS study stated that it may be difficult to detect radiation effect in heavy smokers using an epidemiological approach because of the overwhelming effect of smoking on lung cancer risk in this group [10]. As there would be a large uncertainty in the radiation risk assessment for heavy smokers, it cannot be stated that the LARs of smokers decrease as the number of cigarettes per day increases when the smokers are exposed to a radiation of ≥300 mGy.
3) Results of LAR, AF, and PAF
Table 5 lists the LARs, AF, and PAF of Japanese smokers exposed to radiation doses between 0 and 1,000 mGy. The cause contributing to the risk of developing lung cancer is also shown separately as LBR, radiation-only, smoking-only, and the joint effect of radiation and smoking, respectively. The average value shown in Table 1 was used for smoking risk information.
In Table 5, AF is the fraction of cases estimated to be attributable to that exposure over the total number of cases in that category [6], and PAF is the proportional reduction in population disease or mortality that would occur if exposure to a risk factor were reduced to an alternative ideal exposure scenario (e.g., no smoking) [18]. In Table 5, PAF is calculated by Equation (17) using SR by gender and relative risk (RR) for each exposed level i. In this paper, the exposed level i implies the combination of smoking and radiation dose. RR is defined by dividing the LAR of nonsmoker’s baseline risk by the LAR of total.
where PAFi is the PAFs at exposure level i, and RRi is the RRs at radiation and smoking exposed level i.
First, in comparison, LARs were larger for males than for females up to 500 mGy but were larger for females than for males up to 1,000 mGy, demonstrating that the LAR of females increases and surpasses that of males. This is because females have a markedly greater risk due to the joint effects of radiation and smoking than males.
Second, when comparing AF, the AF of smoking risk was largest in males. In females, the largest AF was also obtained for smoking risk up to 500 mGy. However, the AF obtained at 1,000 mGy demonstrated that the AF of the radiation–smoking interaction risk exceeded that of smoking risk in females. Furthermore, with exposure from 100 to 1,000 mGy, females have a higher AF due to the greater joint effect of radiation and smoking than males. This is one reason why females have a higher ERR per unit dose than males. Moreover, as the average number of cigarettes per day was 12.7 for Japanese females and 16.2 for Japanese males (Table 1), the multiplicative effect due to the number of cigarettes per day in females was larger than that in males. Based on these results, the higher the radiation dose, the higher the LAR and the AF of the joint effect of radiation and smoking in females than in males.
Third, the PAF for smoking was 60% for males and 30% for females. In contrast, the PAF calculated from the measured values [17] was 55.8% (51.2%–60.0%) for male and 16.4% (12.0%–20.5%) for female (current smoker only, ex-smoker not included) [18]. Therefore, the PAF that we calculated was close to that of previous studies. The reason for the difference in PAF values is that the smoking rate is measured in different years. The target population of this paper is that of 2017, whereas the target population of previous studies was that present during the 1980s and early 1990s.
4) Sensitivity analysis: comparison between the GM-ERR model and other ERR modelsIn this session, a sensitivity analysis of radiation and smoking to the ERR models was performed to evaluate the joint effects of radiation and smoking. The GM-ERR models were compared with other ERR models that include the simple additive (SA)-ERR model, the generalized additive (GA)-ERR model, and the simple multiplicative (SM)-ERR model, as described in the study of Cahoon et al. [10]. Three equations of these ERR models [10] are shown below. The values of the parameters in each ERR model were set as the values used by Cahoon et al. [10].
The SA-ERR model:
The GA-ERR model:
The SM-ERR model:
Figs. 6 and 7 show the LARs of smokers at a range of total exposure doses between 100 and 1,000 mGy for each ERR model. In the case of 100 mGy (Figs. 6A and 7A), the highest value in the 10 cigarettes per day group was not apparent in the GM-ERR model for either males or females, indicating no significant difference in these results between the GM-ERR model and other ERR models. Furthermore, when the radiation dose was ≥200 mGy, the result of the GM-ERR model for both males and females was larger than that of other ERR models at approximately c=10.
By investigating the results of other ERR models in Figs. 6 and 7, the SA-ERR and SM-ERR models increased monotonically with respect to the number of cigarettes per day. Next, the results of the SA-ERR model and the SM-ERR model moved apart as the radiation dose increased. This difference in LAR was owing to the term “ERRs×ERRR” in Equation (20). In the GA-ERR model, a peak was observed near c=10 when the radiation dose was 1,000 mGy. However, the magnitude of the peak was smaller than that in the GM-ERR model. This was because f (c) of the GA-ERR model affected only ERRR (Equation [19]), whereas f (c) affected not only ERRR, but also ERRs in Equation (11) of the GM-ERR model.
5) Discussion of the joint effect of smoking and radiationIn this section, we discuss the application of the GM-ERR model for smokers. We also estimated the radiation doses at which the joint effect of smoking and radiation was negligible. To discuss this issue, the difference between the LAR of the GM-ERR model and SA-ERR model was calculated as the ratio of this difference to the LAR of SA-ERR, as shown in in Fig. 8.
Based on the baseline risk estimation of smokers in Fig. 2, the ratios between the fluctuation value of the LAR of smokers due to the standard deviation of cigarettes per day and the average LAR of smokers were approximately ±15% for males and ±22% for females.
If the difference in these ERR models was not significant when the LAR was low within the variation rate due to the standard deviation of the number of cigarettes per day, the radiation dose at which the GM-ERR model replaced the SA-ERR model for both males and females was <200 mGy.
Based on these results, it is apparent that the SA-ERR model can be used in place of the GM-ERR model if the radiation dose is <200 mGy. Therefore, the SA-ERR model of lung cancer risk can be used to evaluate the radiation risk of a population exposed to a low dose.
In Part 2, we discussed the joint effect of smoking and radiation and then suggested that the SA-ERR model can be used in place of the complex GM-ERR model. The optimum effect of around 10 cigarettes per day had been observed in the LSS. The biological mechanism remains unclear. The effect of smoking on lung cancer could be remarkably larger than that of radiation exposure, and this would cause statistical fluctuation. In heavy smokers with ≥10 cigarettes per day, recognizing the radiation-related risk alone will be misleading for preventing health risk. The present study has suggested a significant decrease in nonsmokers regarding the current radiation risk that was estimated using the baseline rate of lung cancer incidence reported by the standard cancer data for population, including smokers.
6) Overall discussionIn Part 1, the baseline risk of nonsmokers was estimated from the baseline risk of the mixed population. In Part 2, the application of the GM-ERR model that expresses the joint effect of radiation and smoking was discussed. The GM-ERR model can be approximated by the SA-ERR model at low doses (several hundred mGy). In this session, we discuss the risk assessment of the lung cancer incidence due to radiation exposure, including the discussion points in Part 1 and Part 2.
Fig. 9 shows the LAR for smoking populations exposed to 100 mGy. Two types of ERR models, a GM-ERR model and an SA-ERR model, were used for comparison. For baseline risk, nonsmokers and the mixed population were used, respectively. The difference in baseline risk in this session depends on whether the baseline λ used to calculate the LAR in Equation (5) is λn of nonsmokers or λm of the mixed population.
According to Fig. 9, the LAR of males is significantly reduced when the baseline risk is changed from mixed population to nonsmokers (average 57% down), whereas the LAR of females showed no significant change (average 26% down). This tendency was similar for the GM-ERR model and the SA-ERR model. It was suggested that the smoking rate among Japanese females was so low that there was no significant difference between the baseline data of the mixed population and nonsmokers. In the future, the smoking rate will decrease with years, and thus the risk assessment even using the mixed population will get closer to that using nonsmoker baseline data.
It became evident that the use of mixed population baseline risk overestimates the LAR of lung cancer due to low-dose radiation exposure in Japanese males. Further investigation will be required to evaluate the radiation risk of other cancers, such as stomach cancer, with consideration of smoking habit. Current LSS studies of solid cancers provide analyses of radiation effects with consideration of smoking history [6]. Risk assessment of nonsmokers must explore how smoking adjusted ERR will be used.
ConclusionThis study has discussed the lifetime risk of lung cancer incidence due to radiation exposure in a Japanese population with consideration given to smoking habit. This paper consisted of two parts. In Part 1, the lung cancer baseline risk of Japanese nonsmokers was estimated. In Part 2, the impact of the joint effect of radiation and smoking was assessed, followed by a discussion on the availability of the GM-ERR model at a low dose.
In Part 1, a simple method of lung cancer baseline risk for nonsmokers from the baseline risk of a mixed population, including smokers, using smoking habit information and the smokers’ ERR model was proposed.
The LBRs from all adults to nonsmokers decreased by 54% (range, 44%–60%) for males and 24% (range, 18%–29%) for females. Based on a sensitivity analysis considering the birth cohort effects, including ex-smokers, it was found that the effects of birth cohort and ex-smokers are less sensitive to the baseline risk estimation of nonsmokers than the number of cigarettes per day.
In Part 2, the lifetime risk of lung cancer incidence was assessed using the GM-ERR model. The LARs of smokers exposed to differing radiation doses and number of cigarettes per day were evaluated. Moreover, a sensitivity analysis of radiation and smoking by other types of ERR models was performed to evaluate the application of the GM-ERR model to the Japanese population. Based on this discussion, it is apparent that the SA-ERR model can be used in place of the GM-ERR model if the radiation dose is <200 mGy.
Finally, our study has shown that the use of the cancer baseline statistics of the mixed population without distinction of smokers and nonsmokers overestimates the risk assessment of lung cancer for nonsmokers by a factor of two in Japanese males.
AcknowledgementsWe would like to thank Shogo Takahara and Tomoyuki Sugiyama of Japan Atomic Energy Agency for supporting us to conduct this research.
NotesAuthor Contribution Conceptualization: Shimada K, Kai M. Data curation: Shimada K. Formal analysis: Shimada K. Methodology: Shimada K. Project administration: Shimada K, Kai M. Visualization: Shimada K. Writing - original draft: Shimada K. Writing - review & editing: Shimada K, Kai M. Investigation: Shimada K. Resources: Shimada K. Software: Shimada K. Supervision: Kai M. Validation: Shimada K. References1. The 2007 Recommendations of the International Commission on Radiological Protection: ICRP publication 103. Ann ICRP. 2007;37:1-332.
2. González AJ, Akashi M, Boice JD Jr, Chino M, Homma T, Ishigure N, et al. Radiological protection issues arising during and after the Fukushima nuclear reactor accident. J Radiol Prot. 2013;33:497-571.
3. Cucinotta FA, Chappell LJ, Kim MH, Wang M. Radiation carcinogenesis risk assessments for never-smokers. Health Phys. 2012;103:643-651.
4. Tirmarche M, Harrison JD, Laurier D, Paquet F, Blanchardon E, Marsh JW, et al. ICRP Publication 115: lung cancer risk from radon and progeny and statement on radon. Ann ICRP. 2010;40:1-64.
5. Cancer and Disease Control Division, Ministry of Health Labour and Welfare. Cancer incidence of Japan [Internet]. Tokyo, Japan, Ministry of Health Labour and Welfare; 2017 [cited 2021 May 1]. Available from: https://ganjoho.jp/en/professional/statistics/table_download.html
6. Grant EJ, Brenner A, Sugiyama H, Sakata R, Sadakane A, Utada M, et al. Solid cancer incidence among the life span study of atomic bomb survivors: 1958–2009. Radiat Res. 2017;187:513-537.
7. Cancer Information Service, National Cancer Center. Cancer registry and statistics [Internet]. Tokyo, Japan, National Cancer Center; 2019 [cited 2021 May 1]. Available from: http://ganjoho.jp/regstat/statistics/dl/index.html
8. Egawa H, Furukawa K, Preston D, Funamoto S, Yonehara S, Matsuo T, et al. Radiation and smoking effects on lung cancer incidence by histological types among atomic bomb survivors. Radiat Res. 2012;178:191-201.
9. Furukawa K, Preston DL, Lonn S, Funamoto S, Yonehara S, Matsuo T, et al. Radiation and smoking effects on lung cancer incidence among atomic bomb survivors. Radiat Res. 2010;174:72-82.
10. Cahoon EK, Preston DL, Pierce DA, Grant E, Brenner AV, Mabuchi K, et al. Lung, laryngeal and other respiratory cancer incidence among Japanese atomic bomb survivors: an updated analysis from 1958 through 2009. Radiat Res. 2017;187:538-548.
11. Ministry of Health Labor and Welfare. National Health and Nutrition Examination Survey 2017 [Internet]. Tokyo, Japan, Ministry of Health Labor and Welfare; 2018 [cited 2021 May 1]. Available from: https://www.mhlw.go.jp/content/10904750/000351576.pdf
12. Japan Health Promotion & Fitness Foundation. The latest tobacco information (JT Japan smoking rates survey) [Internet]. Tokyo, Japan, Japan Health Promotion & Fitness Foundation; 2020 [cited 2021 May 1]. Available from: http://www.health-net.or.jp/tobacco/product/pd090000.html
13. US Environmental Protection Agency. EPA radiogenic cancer risk models and projections for the US population. Washington, DC, US Environmental Protection Agency. 2011.
14. National Research Council. Health risks from exposure to low levels of ionizing radiation (BEIR VII Phase 2). Washington, DC, National Academies Press. 2006.
15. Ministry of Health Labor and Welfare. Outline of vital statistics in Japan [Internet]. Tokyo, Japan, Ministry of Health Labor and Welfare; c2020 [cited 2021 May 1]. Available from: https://www.mhlw.go.jp/english/database/db-hw/outline/index.html
16. Thun MJ, Hannan LM, Adams-Campbell LL, Boffetta P, Buring JE, Feskanich D, et al. Lung cancer occurrence in never-smokers: an analysis of 13 cohorts and 22 cancer registry studies. PLoS Med. 2008;5:e185.
Fig. 1The observed age-specific rates of lung cancer incidence among Japanese males and females in 2017, the estimated lung cancer incidence rates of nonsmokers, and the observed lung cancer incidence rates of nonsmoking females. 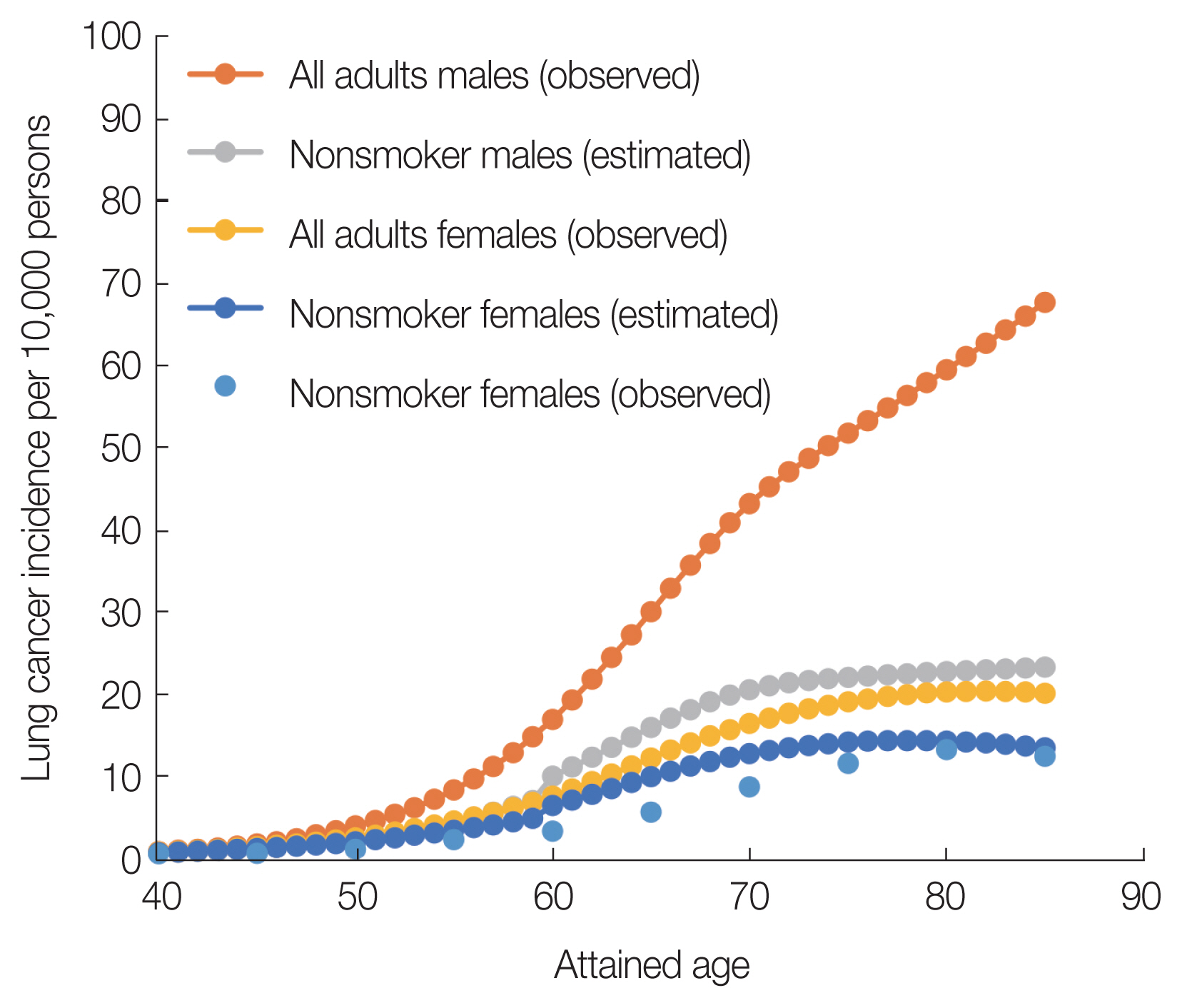
Fig. 3Sensitivity analysis of nonsmokers’ baseline risk estimation considering the cohort effects (birth year effects and ex-smoker information). 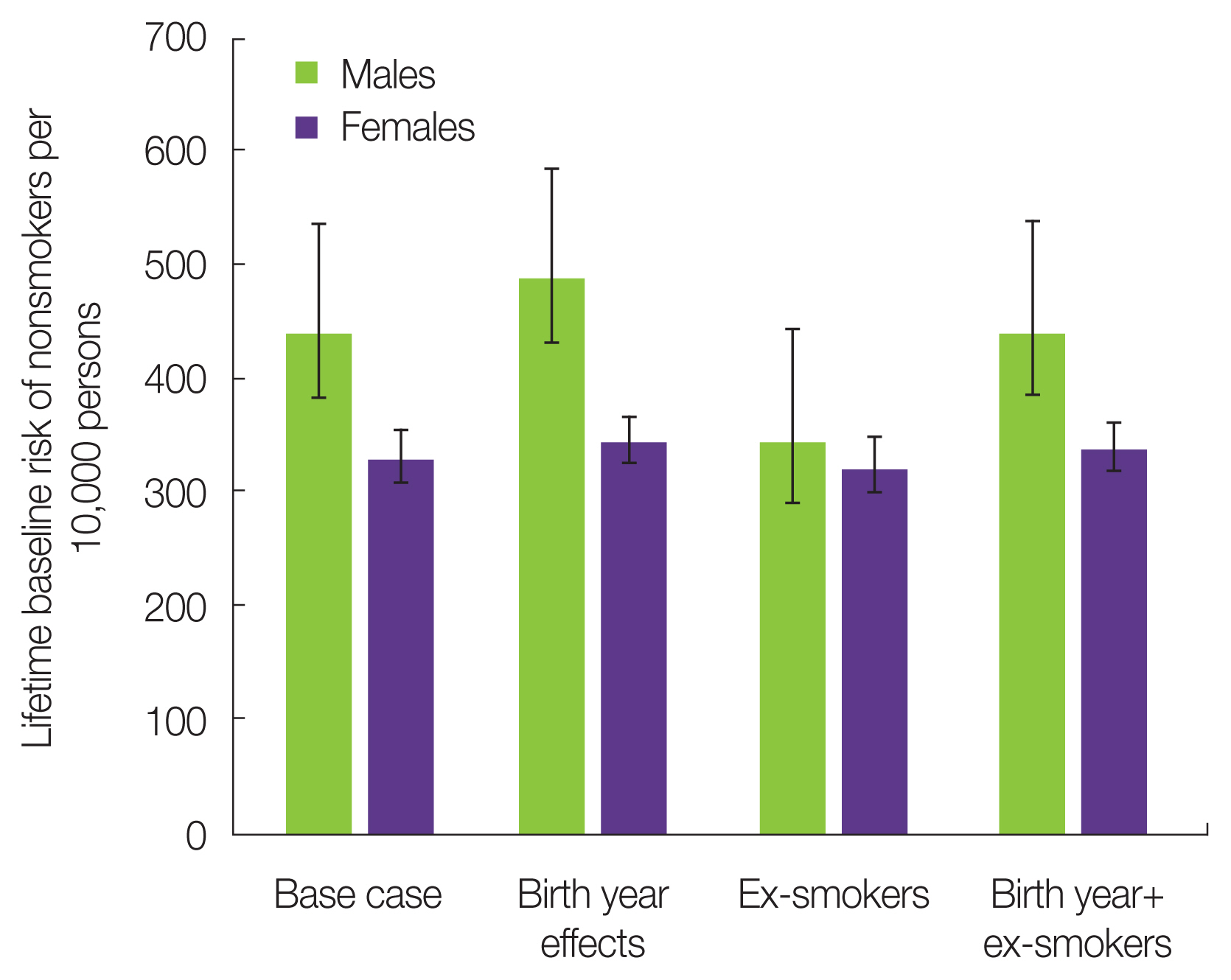
Fig. 4Lifetime attributable risk of lung cancer incidence per 10,000 individuals by age at exposure (100 mGy). 
Fig. 5Lifetime attributable risk due to lung cancer incidence in Japanese who smoke (changes due to total exposure dose and number of cigarettes per day): (A) males and (B) females. 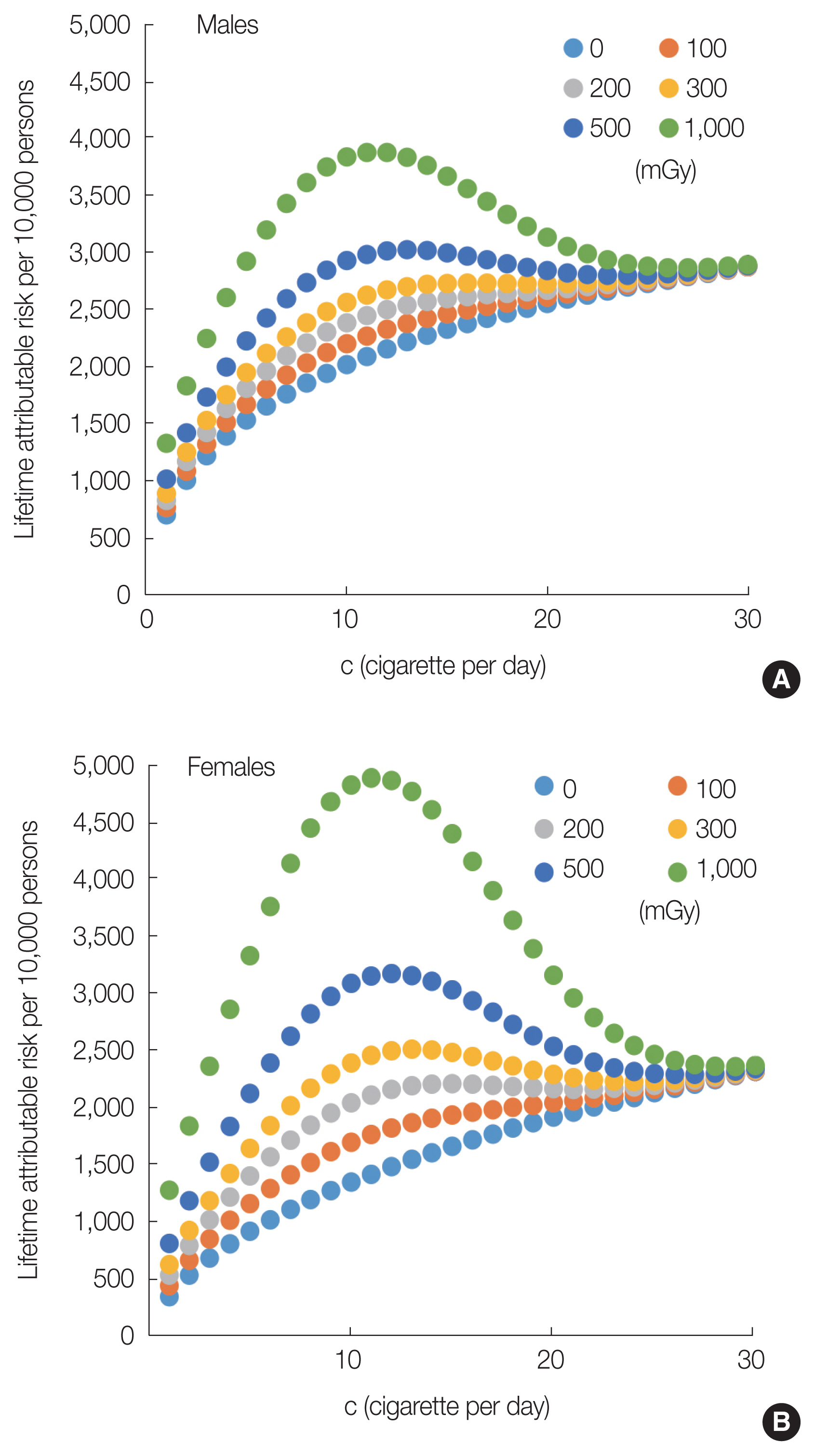
Fig. 6Lifetime attributable risks for male Japanese smokers exposed to 100 to 1,000 mGy of radiation using the GM-, GA-, SA-, and SM-ERR models: (A) 100 mGy, (B) 200 mGy, (C) 300 mGy, (D) 500 mGy, and (E) 1,000 mGy. GM, generalized multiplicative; GA, generalized additive; SA, simple additive; SM, simple multiplicative; ERR, excess relative risk. 
Fig. 7Lifetime attributable risks for female Japanese smokers exposed to 100 to 1,000 mGy of radiation using the GM-, GA-, SA-, and SM-ERR models. (A) 100 mGy, (B) 200 mGy, (C) 300 mGy, (D) 500 mGy, and (E) 1,000 mGy. GM, generalized multiplicative; GA, generalized additive; SA, simple additive; SM, simple multiplicative; ERR, excess relative risk. 
Fig. 8Ratio of the difference between the lifetime attributable risk of the GM-ERR model and the SA-ERR model compared with the SA-ERR model value for 10 cigarettes per day. GM, generalized multiplicative; SA, simple additive; ERR, excess relative risk. 
Fig. 9Lifetime attributable risks for Japanese male and female smokers exposed to 100 mGy of radiation using the GM- and SA-ERR models and nonsmokers baseline risk (λn) and the mixed-population (λm). Error bar represents the standard deviation of cigarettes par day in Table 1. GM, generalized multiplicative; SA, simple additive; ERR, excess relative risk. 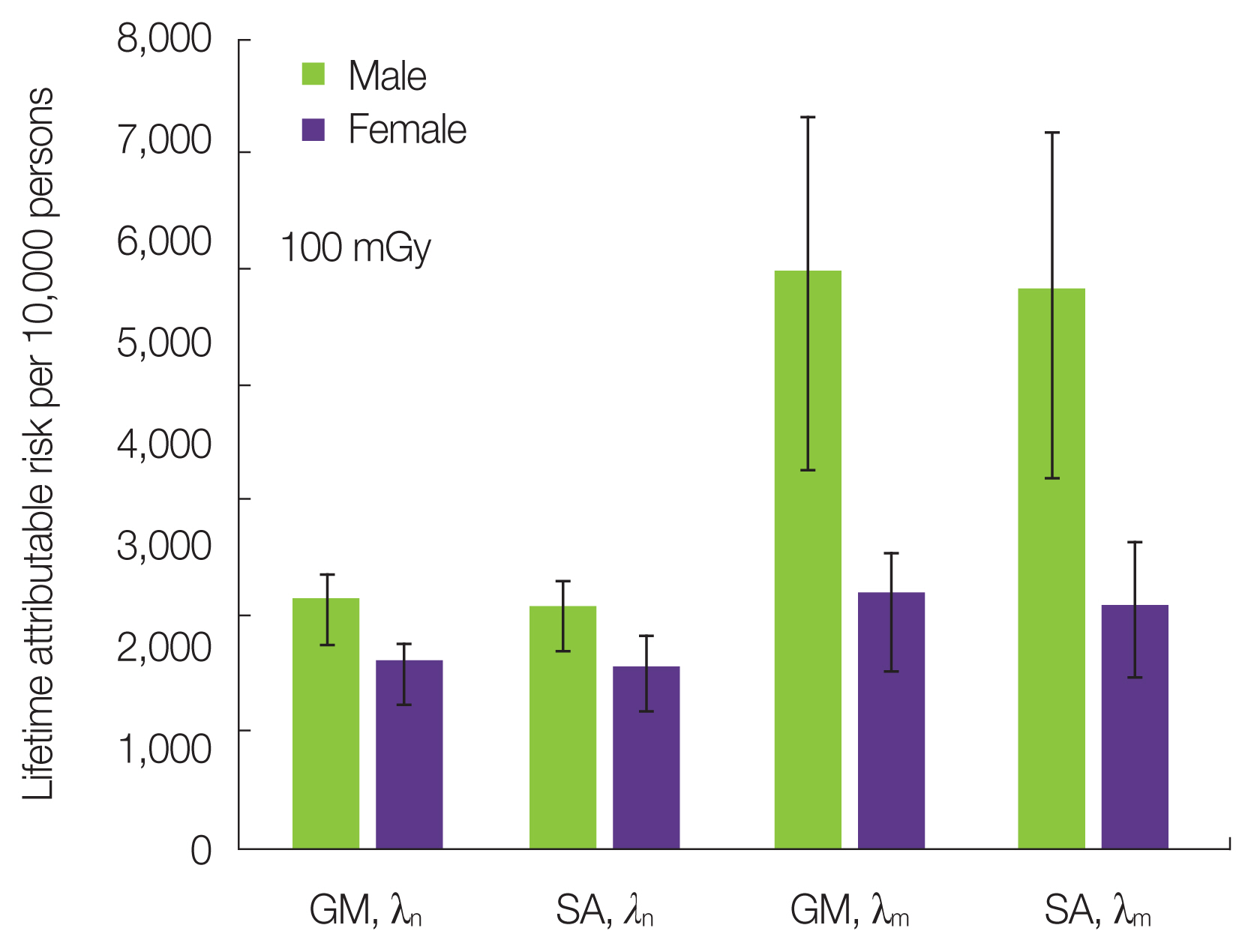
Table 1Data of Smoking Habits of the Japanese Population in 2017
Table 2Annual Trend of Smoking Rates (%) in Japanese Males and Females Data from the Japan Health Promotion & Fitness Foundation [12]. Table 3Quitting Rate of Smoking for Each Age, Current Smoking, and Nonsmoking Rates among Japanese Males and Females in 2017 (unit: %) Table 4The Lifetime Attributable Risk of Lung Cancer Incidence for Age 20–60 Years
Table 5Lifetime Attributable Risks (LAR), Attributable Fractions (AF), and Population Attributable Fractions (PAF) of Japanese Males and Females
|
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||